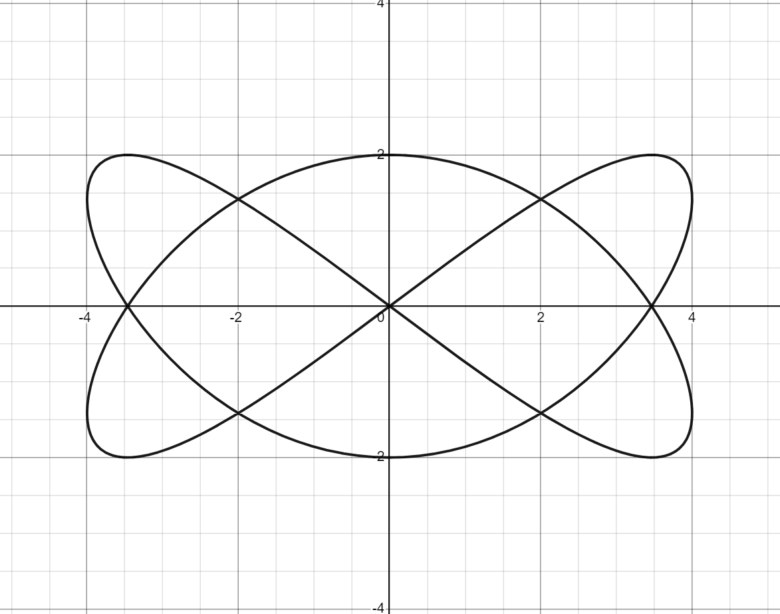
Parametric equations are a fundamental tool in mathematics that describe the motion of objects and the behavior of systems in a way that’s both elegant and powerful. The history of parametric equations is a story of mathematical innovation, driven by the quest to describe complex phenomena in a simpler, more systematic manner. Let’s delve into the rich history of parametric equations and explore their evolution from ancient times to modern mathematics.
The origins of parametric equations can be traced back to ancient Greek mathematics, where astronomers used geometric constructions to model the motion of celestial bodies. One of the earliest examples of parametric equations can be found in the work of Apollonius of Perga, a Greek geometer who lived in the 3rd century BC. Apollonius introduced the concept of epicycles to explain the retrograde motion of planets, using parametric equations to describe the paths of these celestial bodies.

However, it wasn’t until the Renaissance that parametric equations began to take shape in their modern form. In the 17th century, Johannes Kepler used parametric equations to describe the motion of planets around the sun in his famous laws of planetary motion. Kepler’s work laid the foundation for the development of parametric equations as a powerful tool in the study of dynamics and motion.
The true breakthrough in the development of parametric equations came in the 17th century with the work of Pierre de Fermat and René Descartes. Fermat and Descartes independently developed the concept of coordinate geometry, which allowed them to describe geometric shapes using algebraic equations. This laid the groundwork for the modern notation of parametric equations, where two separate equations are used to describe the x and y coordinates of a point in terms of a third variable, typically time.

The use of parametric equations continued to evolve in the 18th and 19th centuries, with mathematicians such as Leonhard Euler and Joseph-Louis Lagrange making significant contributions to their development. Euler, in particular, used parametric equations to study the motion of fluids and the behavior of vibrating strings, laying the foundation for the field of mathematical physics.
In the 20th century, parametric equations found applications in a wide range of fields, from engineering to computer graphics. The advent of computers made it possible to solve complex parametric equations quickly and efficiently, leading to their widespread use in scientific research and technological innovation.
Today, parametric equations continue to play a crucial role in mathematics and its applications. They are used to describe the motion of particles in physics, the behavior of populations in biology, and the shapes of objects in computer graphics. Their elegant simplicity and versatility make them a valuable tool for mathematicians and scientists alike, allowing them to describe complex phenomena in a clear and concise manner.
In conclusion, the history of parametric equations is a testament to the power of mathematics to describe the world around us. From ancient astronomers to modern scientists, mathematicians have used parametric equations to unlock the secrets of the universe and push the boundaries of human knowledge. As we continue to explore the mysteries of the cosmos, parametric equations will undoubtedly remain a vital tool in our quest for understanding.