The History of Sine and Cosine Functions
Sine and cosine functions are fundamental to trigonometry, a branch of mathematics that deals with the relationships between angles and sides of triangles. These two functions are not only key to solving geometric problems but also play a vital role in many areas of science, including physics, engineering, and astronomy. The development of sine and cosine functions is deeply rooted in the history of mathematics, evolving over centuries and across different cultures.
The Origins of Trigonometry
Trigonometry as a field dates back to ancient civilizations. The study of the relationships between the angles and sides of triangles emerged from practical needs such as astronomy, surveying, and construction. The ancient Egyptians and Babylonians made early advancements in geometry and measurements of angles, but the formalization of trigonometry, and by extension the sine and cosine functions, began in ancient Greece and India.
Ancient Greece and Hipparchus
The earliest known systematic use of trigonometry comes from ancient Greece, particularly through the work of Hipparchus of Nicaea (circa 190–120 BCE), often regarded as the “father of trigonometry.” Hipparchus compiled a trigonometric table that used the chord function, which is related to the modern sine function but expressed as the length of a chord subtended by an angle in a circle. Hipparchus’s chord tables were constructed for a circle with a fixed radius, and they were essential for calculating astronomical positions and movements of celestial bodies.
The chord function, however, was a precursor to the sine function, not the sine itself. The concept of sine, as we know it today, began to take shape more fully in the work of Indian mathematicians a few centuries later.
Indian Contributions to Trigonometry: The Birth of the Sine Function
India played a crucial role in the development of trigonometric functions, particularly the sine function. The Sanskrit word for sine, “jya” (or “jiva”), became the foundation of the modern term “sine.” The sine function as a ratio of the length of the opposite side to the hypotenuse in a right triangle was first formalized in Indian mathematical texts.
Aryabhata and the Aryabhatiya
One of the most significant figures in the history of trigonometry was Aryabhata (476–550 CE), an Indian mathematician and astronomer. In his seminal work Aryabhatiya, Aryabhata introduced a form of the sine function, which he referred to as “ardha-jya” (half-chord). Aryabhata compiled trigonometric tables using this concept, which later spread throughout the Islamic world and Europe.
Aryabhata’s sine tables were essential for solving astronomical problems, such as determining the positions of planets and stars. His work laid the foundation for future generations of mathematicians and astronomers in both the East and West.
Bhaskara I and the Development of Trigonometry
Another significant Indian mathematician, Bhaskara I (circa 600–680 CE), further developed Aryabhata’s work on trigonometry. Bhaskara introduced an approximation formula for the sine function that was more accurate than earlier attempts. His efforts were vital in improving the precision of trigonometric calculations, especially for astronomical observations.
The Islamic Golden Age: Transmission and Transformation of Sine and Cosine
During the Islamic Golden Age (8th–14th centuries), the knowledge of Indian trigonometry was preserved, expanded, and transformed by scholars in the Islamic world. These mathematicians translated Indian texts into Arabic and made their own contributions to the study of trigonometry, including the formalization of the cosine function.
Al-Battani and the Cosine Function
Al-Battani (circa 858–929 CE), a prominent astronomer and mathematician, is known for his extensive work on trigonometry. He introduced the cosine function, which is analogous to the sine function but relates to the adjacent side of a triangle rather than the opposite side. Al-Battani compiled detailed trigonometric tables that included both sine and cosine values, providing a more comprehensive framework for solving geometric and astronomical problems.
Al-Battani’s contributions were significant in advancing the understanding of trigonometric relationships. His sine and cosine tables, as well as his methods for calculating the movements of celestial bodies, were widely used in the Islamic world and later influenced European astronomers such as Copernicus.
Al-Khwarizmi and Trigonometric Methods
The renowned Persian scholar Al-Khwarizmi (circa 780–850 CE) is primarily known for his contributions to algebra, but he also played a key role in the transmission of trigonometry to the Islamic world. Al-Khwarizmi’s translations of Indian and Greek mathematical texts introduced trigonometric methods to the Arab-speaking world. His work provided the foundation for future Islamic scholars, who would further develop trigonometry and introduce the concepts of sine and cosine to European mathematicians during the Renaissance.
The Renaissance and the Development of Modern Trigonometry
The Renaissance marked a period of renewed interest in classical knowledge and scientific inquiry, which led to significant advancements in trigonometry. European scholars, influenced by the work of ancient Greek and Islamic mathematicians, further developed the concepts of sine and cosine and integrated them into the broader mathematical framework.
Regiomontanus and Trigonometry in Europe
Johannes Müller von Königsberg (1436–1476), commonly known as Regiomontanus, was one of the most influential figures in the development of trigonometry during the Renaissance. His book De Triangulis Omnimodis (On Triangles of All Kinds), published in 1533, was the first comprehensive work on trigonometry in Europe. Regiomontanus formalized the sine and cosine functions and applied them to solve problems related to both plane and spherical triangles.
Regiomontanus’s work had a profound impact on European mathematics and astronomy, and it marked the beginning of the modern approach to trigonometry. His methods were used by astronomers such as Tycho Brahe and Johannes Kepler in their efforts to understand the movements of planets.
The Modern Era: Sine and Cosine in Mathematics and Science
With the formalization of sine and cosine functions in the Renaissance, these concepts became central to the development of modern mathematics and science. Over the centuries, sine and cosine functions have been applied to a wide range of disciplines, from calculus and differential equations to physics and engineering.
Sine and Cosine in Calculus
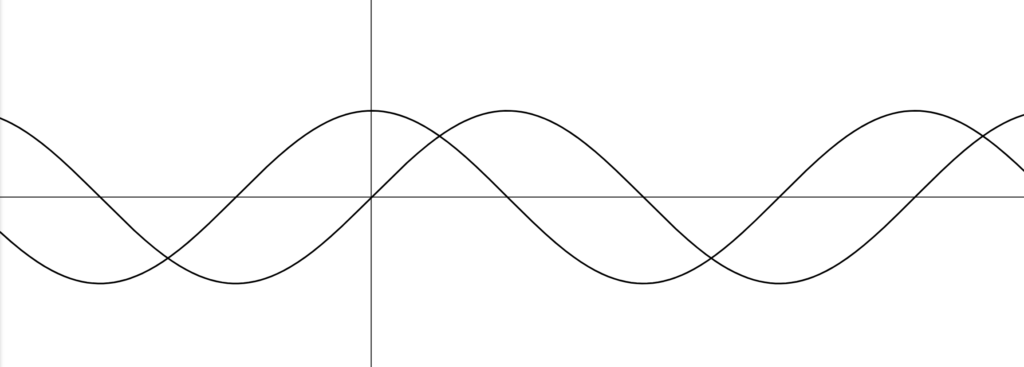
The advent of calculus in the 17th century by Isaac Newton and Gottfried Wilhelm Leibniz opened new avenues for applying sine and cosine functions. These functions play a crucial role in solving differential equations and describing periodic phenomena, such as oscillations and waves. The periodic nature of sine and cosine functions makes them essential for modeling physical systems, including mechanical vibrations, sound waves, and electrical circuits.
Fourier Series and Signal Processing
In the 19th century, the French mathematician Joseph Fourier developed the concept of the Fourier series, which uses sine and cosine functions to represent complex periodic signals. Fourier’s work revolutionized the fields of signal processing, communications, and acoustics. The idea that any periodic function can be expressed as a sum of sine and cosine terms has had a profound impact on modern technology, particularly in areas such as radio transmission, digital signal processing, and image compression.
Sine and Cosine in Modern Physics
In physics, sine and cosine functions are indispensable for describing oscillatory motion, such as the movement of pendulums and springs, as well as wave phenomena, including sound waves, light waves, and electromagnetic radiation. These functions also appear in quantum mechanics and general relativity, where they are used to model the behavior of particles and the curvature of spacetime.
The Lasting Impact of Sine and Cosine Functions
The history of sine and cosine functions is a testament to the power of mathematical concepts to transcend cultural and geographical boundaries. From their origins in ancient Indian and Greek astronomy to their formalization in the Islamic world and their integration into European mathematics, sine and cosine functions have had a profound impact on the development of trigonometry and science.
Today, these functions are essential tools in a wide range of scientific and engineering disciplines, enabling us to understand and model complex systems, from the smallest particles to the largest structures in the universe. The development of sine and cosine functions represents one of the great achievements of human intellectual history, and their applications continue to shape our understanding of the world around us.
Please Visit Our Sponsors:
We only support vendors that we use ourselves in our home. The links below are our own links or affiliate links but know that we use all of these now, or have in the past. As the author/creator of this blog, I also tutor mathematics on Wyzant, sell on Etsy, create content on TpT, and learn Korean on Rosetta Stone.