The Pythagorean Theorem, a fundamental principle in geometry, has stood the test of time as one of the most celebrated and utilized mathematical concepts. Named after the ancient Greek mathematician Pythagoras, this theorem continues to captivate minds and find applications in diverse fields.
Basic Concepts of the Pythagorean Theorem
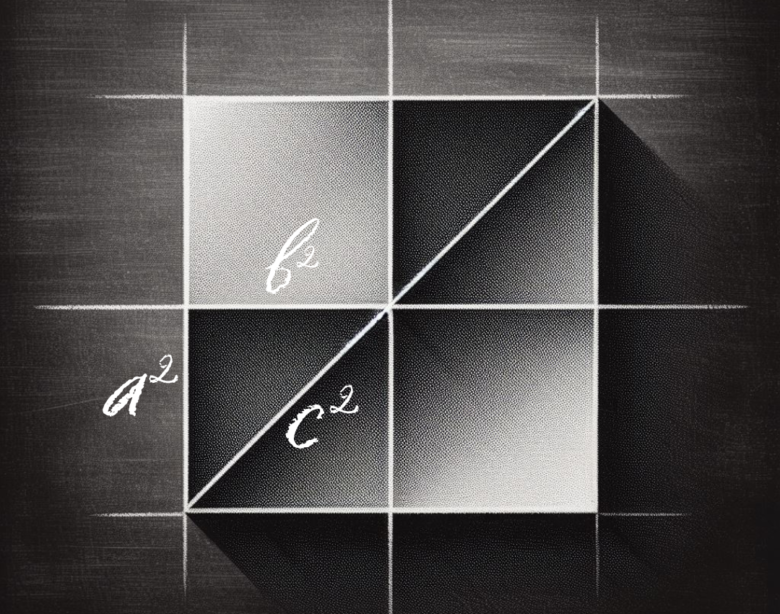
At its core, the Pythagorean Theorem relates to the three sides of a right-angled triangle. It states that in such a triangle, the square of the length of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the lengths of the other two sides. Mathematically, it can be expressed as a\raisebox{0.25em}{$2$} + b\raisebox{0.25em}{$2$} = c\raisebox{0.25em}{$2$} , where c is the length of the hypotenuse, and a and b are the lengths of the other two sides.
History of Discovery
The origin of the Pythagorean Theorem is intertwined with the ancient Greek mathematician Pythagoras, who lived around 570–495 BCE. While the theorem itself existed before Pythagoras, he is credited with its formalization and proof. Legend has it that Pythagoras and his followers discovered the theorem while exploring the relationships between the sides of various right-angled triangles.
Proofs of the Pythagorean Theorem
There are more than 300 accepted proofs for the Pythagorean Theorem.
Historical Proofs by Famous Individuals
- Proof attributed to Pythagoras:
- By legend more than historical fact, we attribute the Pythagoreans for noting that “the sum of the squares of the two legs of a right triangle equal the square of the hypotenuse.”
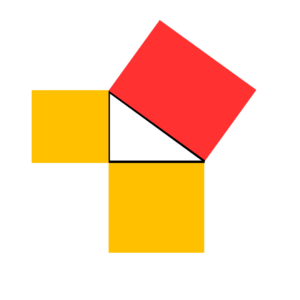
- Proof by Euclid:
- Euclid, the ancient Greek mathematician often referred to as the “father of geometry,” presented a geometric proof in his famous work “Elements.” His elegant proof involves constructing squares on each side of the right-angled triangle.
- Proof by Bhaskara:
- Bhaskara, an Indian mathematician and astronomer from the 12th century, contributed a geometric proof involving a clever dissection of a square.
- Proof in video
- Proof by Leonardo de Vinci:
- Proof by Fermat:
- Pierre de Fermat, a 17th-century mathematician, provided a proof using what is now known as Fermat’s Spiral. This proof adds a captivating visual dimension to the understanding of the theorem.
- Proof by Einstein:
- Per a later publication by Albert Einstein, he described a proof he had found for the theorem when he was twelve years old. Unfortunately, this was not published at the time (as he was twelve) and we do not know if it is a unique proof or if he found a previously published proof independently.
- Proof by President of the United States James A. Garfield:
- The only US President to prove a theorem.
- Proof in video
Conclusion
The Pythagorean Theorem, with its timeless elegance and applicability, remains a cornerstone of geometry. From its ancient origins with Pythagoras to the geometric and algebraic proofs that followed, the theorem has left an indelible mark on the mathematical landscape. As we unravel its mysteries, we gain not only mathematical insights but a profound appreciation for the enduring power of discovery and proof in the world of mathematics.